
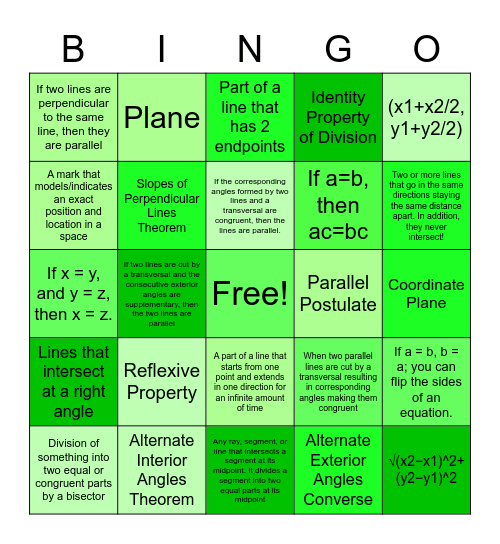
This bingo card has a free space and 24 words: Reflexive Property, Alternate Interior Angles Theorem, If the corresponding angles formed by two lines and a transversal are congruent, then the lines are parallel., Division of something into two equal or congruent parts by a bisector, (x1+x2/2, y1+y2/2), If two lines are perpendicular to the same line, then they are parallel, A mark that models/indicates an exact position and location in a space, Plane, Parallel Postulate, A part of a line that starts from one point and extends in one direction for an infinite amount of time, If a=b, then ac=bc, If x = y, and y = z, then x = z., When two parallel lines are cut by a transversal resulting in corresponding angles making them congruent, If a = b, b = a; you can flip the sides of an equation., Slopes of Perpendicular Lines Theorem, Alternate Exterior Angles Converse, Coordinate Plane, Part of a line that has 2 endpoints, Two or more lines that go in the same directions staying the same distance apart. In addition, they never intersect!, If two lines are cut by a transversal and the consecutive exterior angles are supplementary, then the two lines are parallel, √(x2−x1)^2+(y2−y1)^2, Lines that intersect at a right angle, Identity Property of Division and Any ray, segment, or line that intersects a segment at its midpoint. It divides a segment into two equal parts at its midpoint.
Geometry Bingo | Geometry Bingo | Geometry Bingo | Geometry Bingo | Geometry Bingo
Share this URL with your players:
For more control of your online game, create a clone of this card first.
Learn how to conduct a bingo game.
With players vying for a you'll have to call about __ items before someone wins. There's a __% chance that a lucky player would win after calling __ items.
Tip: If you want your game to last longer (on average), add more unique words/images to it.