f(x)=c Free! Parabola with vertex at origin Symmetry about the y-axis Opens upwards Domain: [0,∞) Domain (-∞, ∞) No Variable Starts at (0,0) Has a point of inflection at the origin Has no real roots Graph decreases as x approaches zero Approaches 0 but never touches the x-axis Free! f(x)=log(x) Horizontal Asymptote f(x)=2^x Range is [0, ∞) Defined for x>0 Straight line through the origin Maximum at the vertex Graph is straight line with slope of 1 f(x)=x^2 No x- intercept Graph passes through (1,0) f(x)=c Free! Parabola with vertex at origin Symmetry about the y-axis Opens upwards Domain: [0,∞) Domain (-∞, ∞) No Variable Starts at (0,0) Has a point of inflection at the origin Has no real roots Graph decreases as x approaches zero Approaches 0 but never touches the x-axis Free! f(x)=log(x) Horizontal Asymptote f(x)=2^x Range is [0, ∞) Defined for x>0 Straight line through the origin Maximum at the vertex Graph is straight line with slope of 1 f(x)=x^2 No x- intercept Graph passes through (1,0)
(Print)
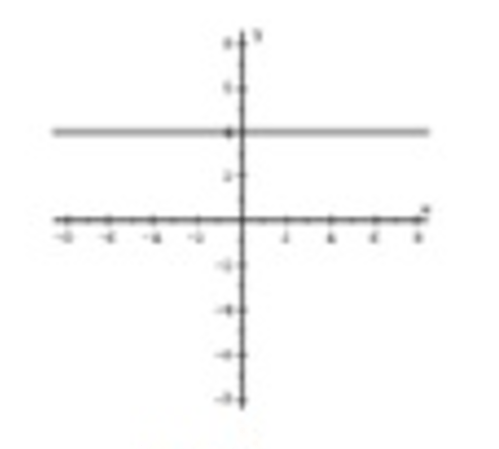
f(x)=c
Free!
Parabola with vertex at origin
Symmetry about the y-axis
Opens upwards
Domain: [0,∞)
Domain (-∞, ∞)
No Variable
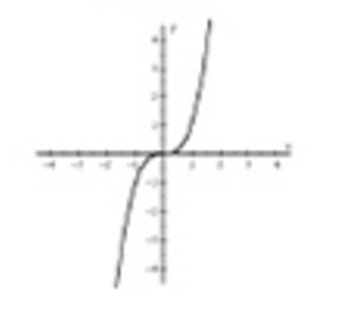
Starts at (0,0)
Has a point of inflection at the origin
Has no real roots
Graph decreases as x approaches zero
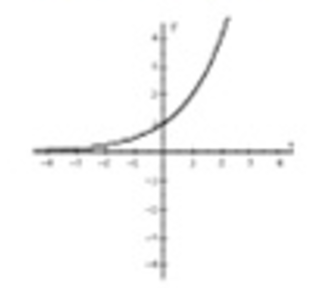
Approaches 0 but never touches the x-axis
Free!
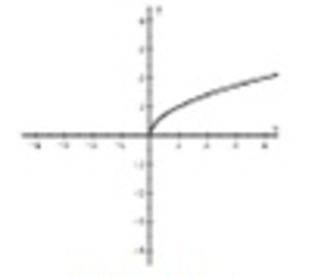
f(x)=log(x)
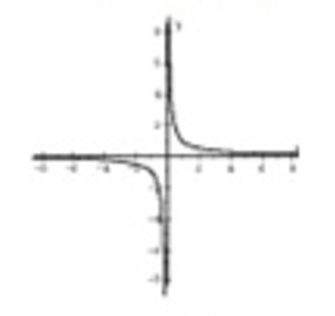
Horizontal Asymptote
f(x)=2^x
Range is [0, ∞)
Defined for x>0
Straight line through the origin
Maximum at the vertex
Graph is straight line with slope of 1
f(x)=x^2
No x-intercept
Graph passes through (1,0)