Unit 6 - Equations and Intersections of Lines

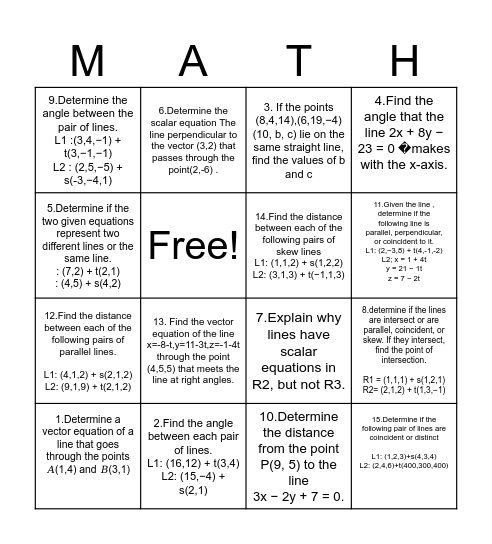
This bingo card has 16 words: 1.Determine a vector equation of a line that goes through the points 𝐴(1,4) and 𝐵(3,1), 2.Find the angle between each pair of lines. L1: (16,12) + t(3,4) L2: (15,−4) + s(2,1), 3. If the points (8,4,14),(6,19,−4) (10, b, c) lie on the same straight line, find the values of b and c, 4.Find the angle that the line 2x + 8y − 23 = 0 �makes with the x-axis., 5.Determine if the two given equations represent two different lines or the same line. : (7,2) + t(2,1) : (4,5) + s(4,2), 6.Determine the scalar equation The line perpendicular to the vector (3,2) that passes through the point(2,-6) ., 7.Explain why lines have scalar equations in R2, but not R3., 8.determine if the lines are intersect or are parallel, coincident, or skew. If they intersect, find the point of intersection. R1 = (1,1,1) + s(1,2,1) R2= (2,1,2) + t(1,3,−1), 9.Determine the angle between the pair of lines. L1 :(3,4,−1) + t(3,−1,−1) L2 : (2,5,−5) + s(-3,−4,1), Free!, 10.Determine the distance from the point P(9, 5) to the line 3x − 2y + 7 = 0., 11.Given the line , determine if the following line is parallel, perpendicular, or coincident to it. L1: (2,−3,8) + t(4,-1,-2) L2; x = 1 + 4t y = 21 − 1t z = 7 − 2t, 12.Find the distance between each of the following pairs of parallel lines. L1: (4,1,2) + s(2,1,2) L2: (9,1,9) + t(2,1,2), 13. Find the vector equation of the line x=-8-t,y=11-3t,z=-1-4t through the point (4,5,5) that meets the line at right angles., 14.Find the distance between each of the following pairs of skew lines L1: (1,1,2) + s(1,2,2) L2: (3,1,3) + t(−1,1,3) and 15.Determine if the following pair of lines are coincident or distinct L1: (1,2,3)+s(4,3,4) L2: (2,4,6)+t(400,300,400).

More like this:

Unit 6 - Equations and Intersections of Lines | Geometry Bingo | Ch 1 Definitions Bingo | Ch 1 Definitions Bingo | Geometry Vocabulary